Z Scores
Courtesy: Wikipedia
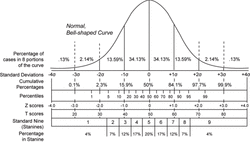
Z Score is a statistical measure that reflects the relative deviance form the median value/standard. It is a pure number and is measured as SD (Standard Deviation) in statistical terms. Z Scores are used in a variety of fields and not just in measuring the nurtitional status of children.
Some of the other statistical options available for measuring relative score are the T Score, Standard Nine and Percentile.
The matter of a relative score becomes all important when there is no agreeable absolute measure for a certain scalar to call it standard or right. For varying measures of a scalar found in a population, we can calculate several different things like a Maximum Value, Minimum Value, Mean (Arithmetic Mean, Geometric Mean or Harmonic Mean), Median, Mode, etc. But what can we say about what it ought to be? Or which is the best? There is no clear answer to this.
This is where the concept of Standard Deviation (SD) comes in. Leaving aside the actual statistical definition of SD, let us just understand it as a measure of deviation of a particular value from the median value. When this is expressed as a positive value and a negative value depending on whether the value is greater than or less than the median, it is called a Z Score. So when the Z Score of an individual measure is 0 (zero), it mean that it is equal to the median.
In the area of Child Health & Nutrition, Z Score is the positive or the negative SD of a particular child with respect to the median of a carefully selected sample or a predetermined population. Z Scores can be of various types depending upon whether the height, weight or both are taken into account. These are better discussed in the following sections.
Therefore, Z Score is a comparative and not an absolute measure in the true sense. However, some extensive studies and research carried out by authoritative bodies like WHO and NCHS/CDC on a global scale have resulted in the setting of certain standardized median values. And today these are used as a reference frame by most nations, most organizations and most researchers.
Please also note that the application of Z Score is not confined to the area of Child Growth & Nutrition and measuring the nutritional status of children. Z Scores can be, and also are used for a variety of purposes including quality control, comparison of examination scores, etc. However, this site will use the term "Z Score" mostly in relation to nutritional status of children.
Some of the other statistical options available for measuring relative score are the T Score, Standard Nine and Percentile.
The matter of a relative score becomes all important when there is no agreeable absolute measure for a certain scalar to call it standard or right. For varying measures of a scalar found in a population, we can calculate several different things like a Maximum Value, Minimum Value, Mean (Arithmetic Mean, Geometric Mean or Harmonic Mean), Median, Mode, etc. But what can we say about what it ought to be? Or which is the best? There is no clear answer to this.
This is where the concept of Standard Deviation (SD) comes in. Leaving aside the actual statistical definition of SD, let us just understand it as a measure of deviation of a particular value from the median value. When this is expressed as a positive value and a negative value depending on whether the value is greater than or less than the median, it is called a Z Score. So when the Z Score of an individual measure is 0 (zero), it mean that it is equal to the median.
In the area of Child Health & Nutrition, Z Score is the positive or the negative SD of a particular child with respect to the median of a carefully selected sample or a predetermined population. Z Scores can be of various types depending upon whether the height, weight or both are taken into account. These are better discussed in the following sections.
Therefore, Z Score is a comparative and not an absolute measure in the true sense. However, some extensive studies and research carried out by authoritative bodies like WHO and NCHS/CDC on a global scale have resulted in the setting of certain standardized median values. And today these are used as a reference frame by most nations, most organizations and most researchers.
Please also note that the application of Z Score is not confined to the area of Child Growth & Nutrition and measuring the nutritional status of children. Z Scores can be, and also are used for a variety of purposes including quality control, comparison of examination scores, etc. However, this site will use the term "Z Score" mostly in relation to nutritional status of children.
Weight for Age Z Score
Keepig in mind the above discussions on the general understanding of Z Scores, a Weight-for-Age Z Score (WAZ) should be understood as the number of standard deviations of the actual weight of a child from the median weight of the children of his/her age as determined from the standard sample. This is prefixed by a positive sign (+) or a negative sign (-) depending on whether the child's actual weight is more than the median weight or less than the median weight.
When the actual weight of a child is exactly equal to the median, the resultant WAZ is 0 (zero). As per the agreed global standards, the following categories can be formed:
1. -1 < WAZ < 0 Normal (Well nourished)
2. -2 < WAZ < -1 Marginally Underweight (Mildly Malnourished)
3. -3 < WAZ < -2 Moderately Underweight (Moderately Malnourished)
4. WAZ < -3 Severely Underweight (Severely Malnourished)
The case of having a below median WAZ is called Underweight and is an indication of malnutrition.
When the actual weight of a child is exactly equal to the median, the resultant WAZ is 0 (zero). As per the agreed global standards, the following categories can be formed:
1. -1 < WAZ < 0 Normal (Well nourished)
2. -2 < WAZ < -1 Marginally Underweight (Mildly Malnourished)
3. -3 < WAZ < -2 Moderately Underweight (Moderately Malnourished)
4. WAZ < -3 Severely Underweight (Severely Malnourished)
The case of having a below median WAZ is called Underweight and is an indication of malnutrition.
Height for Age Z Score
A Height-for-Age Z Score (HAZ) should be understood as the number of standard deviations of the actual height of a child from the median height of the children of his/her age as determined from the standard sample. This is prefixed by a positive sign (+) or a negative sign (-) depending on whether the child's actual height is more than the median height or less than the median weight.
When the actual height of a child is exactly equal to the median, the resultant HAZ is 0 (zero). As per the agreed global standards, the following categories can be formed:
1. -1 < HAZ < 0 Normal (Well nourished)
2. -2 < HAZ < -1 Marginally Stunted (Mildly Malnourished)
3. -3 < HAZ < -2 Moderately Stunted (Moderately Malnourished)
4. HAZ < -3 Severely Stunted (Severely Malnourished)
The case of having a below median HAZ is called Stunting and is an indication of malnutrition.
When the actual height of a child is exactly equal to the median, the resultant HAZ is 0 (zero). As per the agreed global standards, the following categories can be formed:
1. -1 < HAZ < 0 Normal (Well nourished)
2. -2 < HAZ < -1 Marginally Stunted (Mildly Malnourished)
3. -3 < HAZ < -2 Moderately Stunted (Moderately Malnourished)
4. HAZ < -3 Severely Stunted (Severely Malnourished)
The case of having a below median HAZ is called Stunting and is an indication of malnutrition.
Weight for Height Z Score
A Weight-for-Height Z Score (WHZ) should be understood as the number of standard deviations of the actual weight of a child from the median weight of the children of his/her age as determined form the standard sample. This is prefixed by a positive sign (+) or a negative sign (-) depending on whether the child's actual weight is more than the median weight or less than the median weight for the same height.
When the actual weight of a child is exactly equal to the median weight for that height, the resultant WHZ is 0 (zero). As per the agreed global standards, the following categories can be formed:
1. -1 < WAZ < 0 Normal
2. -2 < WAZ < -1 Marginally Wasted
3. -3 < WAZ < -2 Moderately Wasted
4. WHZ < -3 Severely Wasted
The case of having a below median WAZ is called Wasting and is an indication of malnutrition.
When the actual weight of a child is exactly equal to the median weight for that height, the resultant WHZ is 0 (zero). As per the agreed global standards, the following categories can be formed:
1. -1 < WAZ < 0 Normal
2. -2 < WAZ < -1 Marginally Wasted
3. -3 < WAZ < -2 Moderately Wasted
4. WHZ < -3 Severely Wasted
The case of having a below median WAZ is called Wasting and is an indication of malnutrition.
Other Z Scores
There are some other Z scores also like BMI for age Z score, HC for age Z score, etc which are related to pure BMI and HC respectively. But these are not so popular and does not mean much to nutrition specialists. They are rather confortable with absolute BMI and HC measured in cm

